Description
This motif is a variation of Poincaré Kawung, where the Kawung pattern tiled in the Poincaré disk is conformally mapped to a square. Conformal mapping is a function that preserves angles while not necessarily maintaining lengths. Through conformal mapping, the transition between two common shapes, a square and a circle, can be achieved bidirectionally—either from a circle to a square or from a square to a circle. This artistic endeavor is inspired by the pioneering work of Chamberlain Fong in "Analytical Methods for Squaring the Disc".
Math Concept
We can use this formula to map from circle to square:
\( x = Re( \frac{1-i}{-K_e} F(cos ^{-1}(\frac{1+i}{\sqrt{2}} (u + vi)), \frac{1}{\sqrt{2}})) + 1 \)
\( y = Im( \frac{1-i}{-K_e} F(cos ^{-1}(\frac{1+i}{\sqrt{2}} (u + vi)), \frac{1}{\sqrt{2}})) - 1 \)
How to Draw
Any polygon can be transformed into an n-leaf polygon using a straightforward algorithm
Initially, we identify the polygon's center by computing the average of the x-coordinates and y-coordinates of its points. This provides us with the coordinates for the center of the polygon.
Subsequently, a Quadratic Bezier curve is drawn, commencing from the polygon's center as the starting point, utilizing the midpoints of the polygon's vertices as control points, and concluding at the vertices themselves
This process is reiterated by repeating step #3 for each subsequent vertex.
Exhibit
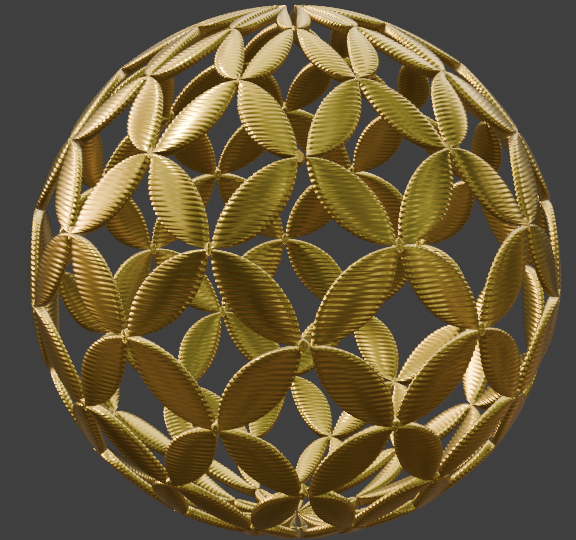
Presenting the Kawung Ball by The Geometry of Batik Team. With the mathematical model in hand, creating a 3D model becomes a straightforward process. The resulting 3D model serves as the foundation for crafting this necklace using the lost wax casting method in our exhibit